This page introduces the typical application of MANOVA and the reporting of the findings.
A brief introduction to the study:
In second language acquisition studies, two competing theories provide guidelines about how to better teach a second language. The 'Intensive Reading' approach maintains that phonics and pronunciation of the words should be the focus of classroom teaching, because human beings start to learn their native language by first learning spoken language. The ‘Extensive Reading' theory, on the other hand, states that language learning process in adults is different from babies. Reading extensively on different topics should be the key of learning a second language.
Suppose a researcher was interested in evaluating the two competing theories by conducting an experiment. Participants at the beginning of the semester were randomly assigned into Intensive, Extensive and Both Methods groups. The Both Methods group spent most classroom time equally in the two methods. At the end of the semester, three tests were administered to all participants: vocabulary, reading comprehension and grammar. The research interest was to statistically examine group difference based on the three reading tests.
Results:
One-Way Multivariate Analysis of Variance (MANOVA) was conducted on three dependent variables: vocabulary, reading comprehension and grammar. The independent variable was group status with three levels: Intensive group ( N = 50), Extensive group (N = 55) and the Both Methods group (N = 48). SPSS version 13 was used to examine outliers, perform MANOVA and produce figures. Specifically, the General Linear Modeling (GLM) approach was used for the MANOVA tests.
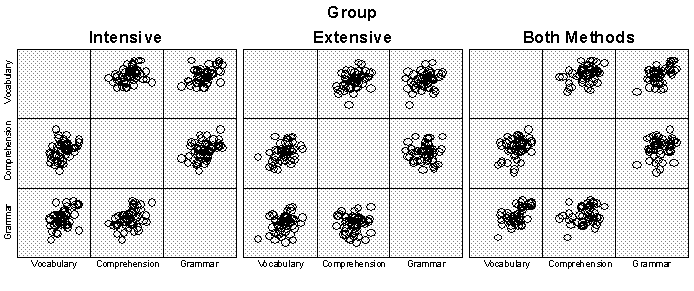
A univariate outlier was defined if a z-score is great than 3 or less than -3 for any dependent variable within a group. One participant in Extensive group was excluded from the analysis as an outlier with an extreme z score on the vocabulary test. No multivariate outlier was found based on the Mahalanobis Distance test. After exclusion of the outlier, univariate normality was examined using the skewness index of dependent variables for each group. Any skewness greater than 1 or less than -1 is an indication of non-normal distribution. All skewness indices in the data were within the proper range, suggesting no serious violation of the normality assumption. Table 1 presents the correlation matrix for three dependent variables showing no extremely high correlation. The linearity assumption was visually examined using a matrix of scatter plots presented in Figure 1. No obvious curvilinear relation was found in the data.
Table 1
Correlation Matrices for Three Dependent Variables Separated by Group
| Intensive | Extensive | Both Methods | ||||
| 2 | 3 | 2 | 3 | 2 | 3 | |
| 1 Vocabulary | .32* | .45** | .39** | .29* | .37** | .60** |
| 2 Comprehension | .42** | .00 | .19 | |||
| 3 Grammar | ||||||
Figure 1
Matrices of Scatter Plots for Three Dependent Variables Separated by Groups showing no curvilinear relation in cells
|
|
MANOVA using Wilks' lambda as the criterion indicated significant group difference in the composite score of the three dependent variables with F (6, 294), p < 0.001. However, the estimated effect size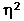 
= 0.1 reflected less than moderate association between groups and the composite of three dependent variables.
To further investigate the group difference in dependent variables, One-Way ANOVA was performed on each dependent variable followed by the Sheffer's Post Hoc test. Results from the ANOVA with the means and standard deviations of the dependent variables for each group are presented in Table 2. It can be seen from Table 2 that there was no significant difference for all three dependent variables between the Intensive and Both Methods groups. However, the Extensive group was significantly lower on vocabulary and grammar tests than the other two groups. For comprehension test, the Extensive group was significantly lower than the Both Methods group, but it was not significantly lower relative to the Intensive group.
Table 2
Means and Standard Deviations for Three Dependent Variables and Mean Comparisons with One-Way ANOVA followed by the Scheffer's Test
| Intensive (N = 50) | Extensive (N = 54) | Both Methods (N = 48) | |
| 1 Vocabulary | 68.10 (15.0)a | 57.80 (15.9)b | 70.38 (16.9)a |
| 2 Comprehension | 64.94 (14.2)a | 61.26 (13.5)ab | 69.65 (15.8)a |
| 3 Grammar | 67.34 (14.8)a | 58.87 (13.6)b | 72.40 (14.0)a |
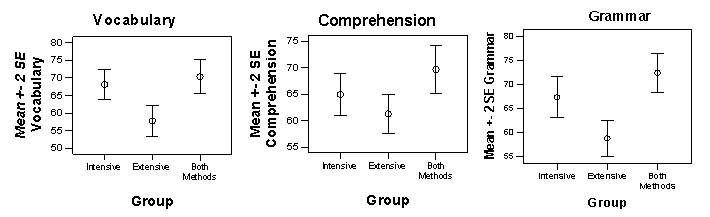
From Table 2, it is clear that the Extensive approach was not as effective as the Intensive approach, but using both methods was not significantly better than only using the Intensive approach. MANOVA comparing the Intensive and Extensive groups was significant with F (3, 100) = 5.0, p < 0.01. However, the MANOVA comparing the Intensive and the Both Methods groups was not significant with F (3, 94) = 1.44, p = 0.237. Results from MANOVA confirmed that the Intensive method was superior over the Extensive method. To aid visual understanding of the findings, Figure 2 presents the means with error bars showing the differences among the groups. It can be seen in Figure 2 that the Extensive group had much lower means in Vocabulary and Grammar tests than the other two groups, but not much lower in the Comprehension test.
Figure 2 Means with Error Bars for the Three Dependent Variables
|
To download the source data, please click here.